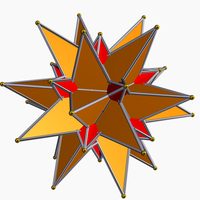
大二十面体
外观
 (按这里观看旋转模型) | ||||
| 类别 | 星形正多面体 星形二十面体 | |||
|---|---|---|---|---|
| 对偶多面体 | 大星形十二面体 | |||
| 识别 | ||||
| 名称 | 大二十面体 | |||
| 参考索引 | U53, C69, W41 | |||
| 鲍尔斯缩写 | gike | |||
| 数学表示法 | ||||
| 考克斯特符号 | ||||
| 施莱夫利符号 | {3,5/2} | |||
| 威佐夫符号 | 5/2 | 2 3 | |||
| 性质 | ||||
| 面 | 20 | |||
| 边 | 30 | |||
| 顶点 | 12 | |||
| 欧拉特征数 | F=20, E=30, V=12 (χ=2) | |||
| 亏格 | 0 | |||
| 组成与布局 | ||||
| 面的种类 | 20个正三角形 | |||
| 面的布局 | 20{3} | |||
| 顶点图 | (35)/2 | |||
| 对称性 | ||||
| 对称群 | Ih, H3, [5,3], (*532) | |||
| 特性 | ||||
| 正、等面、等边、等角 | ||||
| 图像 | ||||
| ||||
在几何学中,大二十面体是一种星形二十面体,由20个正三角形组成,其在非凸均匀多面体被编号为U53、在温尼尔多面体模型被编号为W41,是四种星形正多面体之一,对偶多面体为大星形十二面体。
性质
[编辑]大二十面体共有20个面、30条边和12个顶点[1][2],20个面中,全部都是正三角形,且每个顶点都是5个三角形的公共顶点,但其以类似五角星的方式安排面的位置,使面互相相交,顶点图为五角星,在施莱夫利符号中可以用{3,5/2}来表示,而在考克斯特符号中以![]()
![]()
![]()
![]()
![]()
![]()
![]() 表示。
表示。
顶点座标
[编辑]二面角
[编辑]大二十面体是一种正图形,因此其每个二面角都相等,皆为两个正三角形的棱之交角,其值为五平方根的三分之一之反余弦值[4]:
相关多面体
[编辑]| 名称 | 大星形十二面体 | 截角大星形十二面体 | 大截半二十面体 | 截角大二十面体 | 大二十面体 |
|---|---|---|---|---|---|
| 考式 | |||||
| 图像 | 
|

|

|

|

|
对偶复合体
[编辑]大二十面体与其对偶的复合体为复合大二十面体大星形十二面体。其共有32个面、60条边和32个顶点,其尤拉示性数为4,亏格为-1,有12个非凸面[5],是一种截半二十面体的星形多面体[6]。
|
 从三角形的星状图 |
 从五边形的星状图 |
参考文献
[编辑]- Wenninger, Magnus. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9.
- Coxeter, Harold Scott MacDonald; Du Val, P.; Flather, H. T.; Petrie, J. F. The fifty-nine icosahedra 3rd. Tarquin. 1999. ISBN 978-1-899618-32-3. MR 0676126. (1st Edn University of Toronto (1938))
- H.S.M. Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, 3.6 6.2 Stellating the Platonic solids, pp.96-104
- ^ Uniform Polyhedra 53: great icosahedron. mathconsult.ch. [2016-09-02]. (原始内容存档于2016-03-25).
- ^ great icosahedron. bulatov.org. [2016-09-02]. (原始内容存档于2016-03-27).
- ^ Data of Great Icosahedron. dmccooey.com. [2016-09-02]. (原始内容存档于2016-09-02).
- ^ Kepler-Poinsot Solids: Great Icosahedron. dmccooey.com. [2016-09-02]. (原始内容存档于2016-03-24).
- ^ compound of great stellated dodecahedron and great icosahedron. bulatov.org. [2016-09-02]. (原始内容存档于2015-09-06).
- ^ Weisstein, Eric W. (编). Great Icosahedron-Great Stellated Dodecahedron Compound. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).







