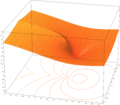
W
0
(
x
)
{\displaystyle W_{0}(x)}
−
1
e
≤
x
≤
4
{\displaystyle -{\frac {1}{e}}\leq x\leq 4}
朗伯W函數 (英語:Lambert W function ,又稱為歐米加函數 或乘積對數 ),是
f
(
w
)
=
w
e
w
{\displaystyle f(w)=we^{w}}
反函數 ,其中
e
w
{\displaystyle e^{w}}
指數函數 ,
w
{\displaystyle w}
複數 。對於任何複數
z
{\displaystyle z}
z
=
W
(
z
)
e
W
(
z
)
.
{\displaystyle z=W(z)e^{W(z)}.}
由於函數
f
{\displaystyle f}
單射 ,因此函數
W
{\displaystyle W}
多值 的(除了0以外)。如果我們把
x
{\displaystyle x}
w
{\displaystyle w}
x
≥
1
e
{\displaystyle x\geq {\frac {1}{e}}}
(
−
1
e
,
0
)
{\displaystyle (-{\frac {1}{e}},0)}
w
≥
−
1
{\displaystyle w\geq -1}
W
0
(
x
)
{\displaystyle W_{0}(x)}
W
0
(
0
)
=
0
{\displaystyle W_{0}(0)=0}
W
0
(
−
1
e
)
=
−
1
{\displaystyle W_{0}(-{\frac {1}{e}})=-1}
[
−
1
e
,
0
)
{\displaystyle [-{\frac {1}{e}},0)}
w
≤
−
1
{\displaystyle w\leq -1}
W
−
1
(
x
)
{\displaystyle W_{-1}(x)}
W
−
1
(
−
1
e
)
=
−
1
{\displaystyle W_{-1}(-{\frac {1}{e}})=-1}
W
−
1
(
0
−
)
=
−
∞
{\displaystyle W_{-1}(0^{-})=-\infty }
朗伯
W
{\displaystyle W}
初等函數 來表示。它在組合數學 中有許多用途,例如樹 的計算。它可以用來解許多含有指數的方程,也出現在某些微分方程 的解中,例如
y
(
t
)
=
a
y
(
t
−
1
)
{\displaystyle y(t)=ay(t-1)}
複平面上的朗伯W函數的函數圖形 朗伯
W
{\displaystyle W\,}
W
(
x
)
=
x
π
∫
0
π
(
1
−
v
cot
v
)
2
+
v
2
x
+
v
csc
v
⋅
e
−
v
cot
v
d
v
,
|
arg
(
x
)
|
<
π
{\displaystyle W(x)={\frac {x}{\pi }}\int _{0}^{\pi }{\frac {\left(1-v\cot v\right)^{2}+v^{2}}{x+v\csc v\cdot e^{-v\cot v}}}{\rm {d}}v,|\arg \left(x\right)|<\pi \,}
W
(
x
)
=
∫
−
∞
−
1
e
−
1
π
ℑ
[
d
d
x
W
(
x
)
]
ln
(
1
−
z
x
)
d
x
{\displaystyle W(x)=\int _{-\infty }^{-{\frac {1}{e}}}{-{\frac {1}{\pi }}}\Im \left[{\frac {\rm {d}}{{\rm {d}}x}}W(x)\right]\ln \left(1-{\frac {z}{x}}\right){\rm {d}}x\,}
若
x
∉
[
−
1
e
,
0
]
,
k
∈
Z
{\displaystyle x\not \in \left[-{\frac {1}{e}},0\right],k\in {\mathbb {Z} }\,}
x
∈
(
−
1
e
,
0
)
,
k
=
1
,
±
2
,
±
3
,
.
.
.
{\displaystyle x\in \left(-{\frac {1}{e}},0\right),k=1,\pm 2,\pm 3,...\,}
W
k
(
x
)
=
1
+
(
ln
x
−
1
+
2
k
π
i
)
e
i
2
π
∫
0
∞
ln
t
−
ln
t
+
ln
x
+
(
2
k
+
1
)
π
i
t
−
ln
t
+
ln
x
+
(
2
k
−
1
)
π
i
⋅
d
t
t
+
1
=
1
+
(
ln
x
−
1
+
2
k
π
i
)
e
i
2
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
+
2
π
(
t
−
ln
t
+
ln
x
)
i
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
{\displaystyle W_{k}(x)=1+\left(\ln x-1+2k\pi {\rm {i}}\right)e^{{\frac {\rm {i}}{2\pi }}\int _{0}^{\infty }\ln {\frac {t-\ln t+\ln x+(2k+1)\pi {\rm {i}}}{t-\ln t+\ln x+(2k-1)\pi {\rm {i}}}}\cdot {\frac {{\rm {d}}t}{t+1}}}=1+\left(\ln x-1+2k\pi {\rm {i}}\right)e^{{\frac {\rm {i}}{2\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}+2\pi \left(t-\ln t+\ln x\right){\rm {i}}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}}\,}
把被積函數的實部和虛部分離出來:
W
k
(
x
)
=
1
+
(
ln
x
−
1
+
2
k
π
i
)
e
i
2
π
∫
0
∞
[
1
2
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
+
i
arctan
2
π
(
t
−
ln
t
+
ln
x
)
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
]
⋅
d
t
t
+
1
{\displaystyle W_{k}(x)=1+\left(\ln x-1+2k\pi {\rm {i}}\right)e^{{\frac {\rm {i}}{2\pi }}\int _{0}^{\infty }\left[{\frac {1}{2}}\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}+{\rm {i}}\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\right]\cdot {\frac {{\rm {d}}t}{t+1}}}}
W
k
(
x
)
=
1
+
(
ln
x
−
1
)
cos
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
−
2
k
π
sin
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
+
i
[
(
ln
x
−
1
)
sin
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
+
2
k
π
cos
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
]
e
1
2
π
∫
0
∞
arctan
2
π
(
t
−
ln
t
+
ln
x
)
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
⋅
d
t
t
+
1
{\displaystyle {}_{W_{k}(x)=1+{\frac {\left(\ln x-1\right)\cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}-2k\pi \sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+{\rm {i}}\left[\left(\ln x-1\right)\sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+2k\pi \cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}\right]}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\cdot {\frac {\rm {{d}t}}{t+1}}}}}}}
W
k
(
x
)
=
u
+
v
i
,
x
=
t
+
s
i
{\displaystyle W_{k}(x)=u+v{\rm {i}},x=t+s{\rm {i}}}
(
u
+
v
i
)
e
u
+
v
i
=
t
+
s
i
{\displaystyle \left(u+v{\rm {i}}\right)e^{u+v{\rm {i}}}=t+s{\rm {i}}}
e
u
(
u
cos
v
−
v
sin
v
)
=
t
,
e
u
(
u
sin
v
+
v
cos
v
)
=
s
{\displaystyle e^{u}\left(u\cos v-v\sin v\right)=t,e^{u}\left(u\sin v+v\cos v\right)=s}
s
=
0
{\displaystyle s=0}
u
=
−
v
cot
v
{\displaystyle u=-v\cot v}
W
k
(
x
)
=
(
1
−
ln
x
)
sin
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
−
2
k
π
cos
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
e
1
2
π
∫
0
∞
arctan
2
π
(
t
−
ln
t
+
ln
x
)
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
⋅
d
t
t
+
1
cot
(
ln
x
−
1
)
sin
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
+
2
k
π
cos
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
e
1
2
π
∫
0
∞
arctan
2
π
(
t
−
ln
t
+
ln
x
)
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
⋅
d
t
t
+
1
+
(
ln
x
−
1
)
sin
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
+
2
k
π
cos
1
4
π
∫
0
∞
ln
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
+
1
)
2
π
2
(
t
−
ln
t
+
ln
x
)
2
+
(
2
k
−
1
)
2
π
2
⋅
d
t
t
+
1
e
1
2
π
∫
0
∞
arctan
2
π
(
t
−
ln
t
+
ln
x
)
(
t
−
ln
t
+
ln
x
)
2
+
(
4
k
2
−
1
)
π
2
⋅
d
t
t
+
1
i
,
{\displaystyle {}_{W_{k}(x)={\frac {\left(1-\ln x\right)\sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}-2k\pi \cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\cdot {\frac {\rm {{d}t}}{t+1}}}}}\cot {\frac {\left(\ln x-1\right)\sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+2k\pi \cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\cdot {\frac {\rm {{d}t}}{t+1}}}}}+{\frac {\left(\ln x-1\right)\sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+2k\pi \cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\cdot {\frac {\rm {{d}t}}{t+1}}}}}{\rm {i}},}}
W
0
(
x
)
=
1
+
(
ln
x
−
1
)
e
−
1
π
∫
0
∞
arg
(
t
−
ln
t
+
ln
x
+
π
i
)
⋅
d
t
t
+
1
,
x
>
0
{\displaystyle W_{0}(x)=1+\left(\ln x-1\right)e^{-{\frac {1}{\pi }}\int _{0}^{\infty }\arg \left(t-\ln t+\ln x+\pi {\rm {i}}\right)\cdot {\frac {\rm {{d}t}}{t+1}}},x>0}
若
x
>
1
e
{\displaystyle x>{\frac {1}{e}}}
W
0
(
x
)
=
1
+
(
ln
x
−
1
)
e
−
1
π
∫
0
∞
arctan
π
t
−
ln
t
+
ln
x
⋅
d
t
t
+
1
{\displaystyle W_{0}(x)=1+\left(\ln x-1\right)e^{-{\frac {1}{\pi }}\int _{0}^{\infty }\arctan {\frac {\pi }{t-\ln t+\ln x}}\cdot {\frac {\rm {{d}t}}{t+1}}}}
由隱函數 的求導法則,朗伯
W
{\displaystyle W\,}
微分方程 :
z
[
1
+
W
(
z
)
]
d
d
z
W
(
z
)
=
W
(
z
)
{\displaystyle z\left[1+W(z)\right]{\frac {\rm {d}}{{\rm {d}}z}}W(z)=W(z)}
z
≠
−
1
e
,
{\displaystyle z\neq -{\frac {1}{e}}\,,}
因此:
d
d
z
W
(
z
)
=
W
(
z
)
z
[
1
+
W
(
z
)
]
{\displaystyle {\frac {\rm {d}}{{\rm {d}}z}}W(z)={\frac {W(z)}{z\left[1+W(z)\right]}}}
z
≠
−
1
e
.
{\displaystyle z\neq -{\frac {1}{e}}\,.}
函數
W
(
x
)
{\displaystyle W(x)\,}
W
(
x
)
{\displaystyle W(x)\,}
w
=
W
(
x
)
{\displaystyle w=W(x)\,}
變量代換 來積分,也就是說
x
=
w
e
w
{\displaystyle x=we^{w}\,}
∫
W
(
x
)
d
x
=
x
[
W
(
x
)
+
1
W
(
x
)
−
1
]
+
C
{\displaystyle \int W(x){\rm {d}}x=x\left[W(x)+{\frac {1}{W(x)}}-1\right]+C}
∫
0
1
W
(
x
)
d
x
=
Ω
+
1
Ω
−
2
≈
0.330366
{\displaystyle \int _{0}^{1}W(x){\rm {d}}x=\Omega +{\frac {1}{\Omega }}-2\approx 0.330366}
其中
Ω
{\displaystyle \Omega }
歐米加常數 。
1
{\displaystyle 1\,}
z
z
z
z
z
.
.
.
=
lim
n
→
∞
(
z
⇈
n
)
=
−
W
(
−
ln
z
)
ln
z
{\displaystyle z^{z^{z^{z^{z^{.^{.^{.}}}}}}}=\lim _{n\to \infty }(z\upuparrows n)=-{\frac {W(-\ln z)}{\ln z}}}
其中
⇈
{\displaystyle \upuparrows }
高德納箭號表示法 。
2
{\displaystyle 2\,}
z
>
0
{\displaystyle z>0\,}
ln
W
(
z
)
=
ln
z
−
W
(
z
)
{\displaystyle \ln W(z)=\ln z-W(z)\,}
W
0
{\displaystyle W_{0}\,}
x
=
0
{\displaystyle x=0\,}
W
0
(
x
)
=
∑
n
=
1
∞
(
−
n
)
n
−
1
n
!
x
n
=
x
−
x
2
+
3
2
x
3
−
8
3
x
4
+
125
24
x
5
−
⋯
{\displaystyle W_{0}(x)=\sum _{n=1}^{\infty }{\frac {(-n)^{n-1}}{n!}}\ x^{n}=x-x^{2}+{\frac {3}{2}}x^{3}-{\frac {8}{3}}x^{4}+{\frac {125}{24}}x^{5}-\cdots }
收斂半徑 為
1
e
{\displaystyle {\frac {1}{e}}\,}
W
(
x
)
+
W
(
y
)
=
W
[
x
y
W
(
x
)
+
x
y
W
(
y
)
]
{\displaystyle W(x)+W(y)=W\left[{\frac {xy}{W(x)}}+{\frac {xy}{W(y)}}\right]\,}
x
>
0
,
y
>
0
{\displaystyle x>0,y>0\,}
實部
ℜ
[
W
(
x
+
y
i
)
]
=
∑
k
=
1
∞
(
−
k
)
k
−
1
k
!
(
x
2
+
y
2
)
k
cos
(
k
arctan
x
y
)
{\displaystyle \Re \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\cos \left(k\arctan {\frac {x}{y}}\right)\,}
x
2
+
y
2
<
1
e
2
{\displaystyle x^{2}+y^{2}<{\frac {1}{e^{2}}}\,}
虛部
ℑ
[
W
(
x
+
y
i
)
]
=
∑
k
=
1
∞
(
−
k
)
k
−
1
k
!
(
x
2
+
y
2
)
k
sin
(
k
arctan
x
y
)
{\displaystyle \Im \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\sin \left(k\arctan {\frac {x}{y}}\right)\,}
x
2
+
y
2
<
1
e
2
{\displaystyle x^{2}+y^{2}<{\frac {1}{e^{2}}}\,}
模長
|
W
(
x
+
y
i
)
|
=
W
(
x
+
y
)
{\displaystyle |W(x+y{\rm {i}})|=W({\sqrt {x+y}})\,}
模角
arg
[
W
(
x
+
y
i
)
]
=
∑
k
=
1
∞
(
−
k
)
k
−
1
k
!
arctan
[
cot
(
k
arctan
x
y
)
]
{\displaystyle \arg \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}\arctan \left[\cot(k\arctan {\frac {x}{y}})\right]\,}
x
2
+
y
2
<
1
e
2
{\displaystyle x^{2}+y^{2}<{\frac {1}{e^{2}}}\,}
共軛值
W
(
x
+
y
i
)
¯
=
∑
k
=
1
∞
(
−
k
)
k
−
1
k
!
(
x
2
+
y
2
)
k
[
cos
(
k
arctan
x
y
)
−
i
sin
(
k
arctan
x
y
)
]
{\displaystyle {\overline {W(x+y{\rm {i}})}}=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\left[\cos \left(k\arctan {\frac {x}{y}}\right)-{\rm {i}}\sin \left(k\arctan {\frac {x}{y}}\right)\right]\,}
x
2
+
y
2
<
1
e
2
{\displaystyle x^{2}+y^{2}<{\frac {1}{e^{2}}}\,}
W
(
−
π
2
)
=
π
2
i
{\displaystyle W\left(-{\frac {\pi }{2}}\right)={\frac {\pi }{2}}i}
W
(
−
ln
2
2
)
=
−
ln
2
{\displaystyle W\left(-{\frac {\ln 2}{2}}\right)=-\ln 2}
W
(
−
1
e
)
=
−
1
{\displaystyle W\left(-{1 \over e}\right)=-1}
W
(
1
)
=
Ω
=
1
∫
−
∞
∞
d
x
(
e
x
−
x
)
2
+
π
2
−
1
≈
0.56714329
…
{\displaystyle W\left(1\right)=\Omega ={\frac {1}{\int _{-\infty }^{\infty }{\frac {{\rm {d}}x}{(e^{x}-x)^{2}+\pi ^{2}}}}}-1\approx 0.56714329\dots \,}
歐米加常數 )
W
(
e
)
=
1
{\displaystyle W(e)=1\,}
W
(
e
e
+
1
)
=
e
{\displaystyle W(e^{e+1})=e\,}
W
(
1
e
1
−
1
e
)
=
1
e
{\displaystyle W\left({\frac {1}{e^{1-{\frac {1}{e}}}}}\right)={\frac {1}{e}}}
W
(
π
e
π
)
=
π
{\displaystyle W({\pi }e^{\pi })=\pi }
W
(
k
ln
k
)
=
ln
k
{\displaystyle W(k{\ln k})={\ln k}}
(
k
>
0
)
{\displaystyle (k>0)}
W
(
i
π
)
=
−
i
π
{\displaystyle W({\rm {i}}\pi )=-{\rm {i}}\pi }
W
(
−
i
π
)
=
i
π
{\displaystyle W(-{\rm {i}}\pi )={\rm {i}}\pi }
W
(
i
cos
1
−
sin
1
)
=
i
{\displaystyle W({\rm {i}}\cos 1-\sin 1)={\rm {i}}}
W
(
−
3
2
π
)
=
−
3
2
π
i
{\displaystyle W(-{\frac {3}{2}}{\pi })=-{\frac {3}{2}}{\pi }{\rm {i}}}
W
(
−
8
7
7
ln
2
)
=
−
32
7
ln
2
{\displaystyle W(-{\frac {\sqrt[{7}]{8}}{7}}{\ln 2})=-{\frac {32}{7}}{\ln 2}}
W
(
−
3
54
ln
3
)
=
−
9
2
ln
3
{\displaystyle W(-{\frac {\sqrt {3}}{54}}{\ln 3})=-{\frac {9}{2}}{\ln 3}}
W
(
−
ln
2
4
)
=
−
4
ln
2
{\displaystyle W(-{\frac {\ln 2}{4}})=-4{\ln 2}}
W
(
−
1
)
=
e
1
2
π
∫
0
∞
1
t
+
1
arctan
2
π
t
−
ln
t
d
t
−
cos
[
1
4
π
∫
0
∞
1
t
+
1
ln
(
t
−
ln
t
)
2
4
π
2
+
(
t
−
ln
t
)
2
d
t
]
+
π
sin
[
1
4
π
∫
0
∞
1
t
+
1
ln
(
t
−
ln
t
)
2
4
π
2
+
(
t
−
ln
t
)
2
d
t
]
−
i
{
π
cos
[
1
4
π
∫
0
∞
1
t
+
1
ln
(
t
−
ln
t
)
2
4
π
2
+
(
t
−
ln
t
)
2
d
t
]
+
sin
[
1
4
π
∫
0
∞
1
t
+
1
ln
(
t
−
ln
t
)
2
4
π
2
+
(
t
−
ln
t
)
2
d
t
]
}
e
1
2
π
∫
0
∞
1
t
+
1
arctan
2
π
t
−
ln
t
d
t
≈
−
0.31813
−
1.33723
i
{\displaystyle W\left(-1\right)={\frac {e^{{\frac {1}{2\pi }}\int _{0}^{\infty }{1 \over t+1}\arctan {2\pi \over t-\ln t}{\rm {d}}t}-\cos \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]+\pi \sin \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]-{\rm {i}}\left\{\pi \cos \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]+\sin \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]\right\}}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }{1 \over t+1}\arctan {2\pi \over t-\ln t}{\rm {d}}t}}}\approx -0.31813-1.33723{\rm {i}}}
W
(
−
ln
k
k
)
=
−
ln
k
{\displaystyle W(-{\frac {\ln k}{k}})=-\ln k}
W
[
−
ln
(
x
+
1
)
x
(
x
+
1
)
1
x
]
=
−
x
+
1
x
ln
(
x
+
1
)
>
,
−
1
<
x
<
0
{\displaystyle W\left[-{\frac {\ln(x+1)}{x(x+1)^{\frac {1}{x}}}}\right]=-{\frac {x+1}{x}}\ln(x+1)>,-1<x<0}
許多含有指數的方程都可以用
W
{\displaystyle W\,}
Y
=
X
e
X
{\displaystyle Y=Xe^{X}\,}
例子1
2
t
=
5
t
{\displaystyle 2^{t}=5t\,}
⇒
1
=
5
t
2
t
{\displaystyle \Rightarrow 1={\frac {5t}{2^{t}}}\,}
⇒
1
=
5
t
e
−
t
ln
2
{\displaystyle \Rightarrow 1=5t\,e^{-t\ln 2}\,}
⇒
1
5
=
t
e
−
t
ln
2
{\displaystyle \Rightarrow {\frac {1}{5}}=t\,e^{-t\ln 2}\,}
⇒
−
ln
2
5
=
(
−
t
ln
2
)
e
−
t
ln
2
{\displaystyle \Rightarrow -{\frac {\ln 2}{5}}=(-\,t\,\ln 2)\,e^{-t\ln 2}\,}
⇒
−
t
ln
2
=
W
k
(
−
ln
2
5
)
{\displaystyle \Rightarrow -t\ln 2=W_{k}\left(-{\frac {\ln 2}{5}}\right)\,}
⇒
t
=
−
W
k
(
−
ln
2
5
)
ln
2
{\displaystyle \Rightarrow t=-{\frac {W_{k}\left(-{\frac {\ln 2}{5}}\right)}{\ln 2}}\,}
更一般地,以下的方程
Q
a
x
+
b
=
c
x
+
d
{\displaystyle Q^{ax+b}=cx+d\,}
其中
Q
>
0
∧
Q
≠
1
∧
c
≠
0
{\displaystyle Q>0\land Q\neq 1\land c\neq 0}
兩邊同乘:
a
c
{\displaystyle {\frac {a}{c}}}
得到:
a
c
Q
a
x
+
b
=
a
x
+
a
d
c
{\displaystyle {\frac {a}{c}}Q^{ax+b}=ax+{\frac {ad}{c}}\,}
同除以:
Q
a
x
{\displaystyle Q^{ax}\,}
得到:
a
c
Q
b
=
(
a
x
+
a
d
c
)
Q
−
a
x
{\displaystyle {\frac {a}{c}}Q^{b}=\left(ax+{\frac {ad}{c}}\right)Q^{-ax}\,}
同除:
Q
a
d
c
{\displaystyle Q^{\frac {ad}{c}}\,}
a
c
Q
b
−
a
d
c
=
(
a
x
+
a
d
c
)
Q
−
(
a
x
+
a
d
c
)
{\displaystyle {\frac {a}{c}}Q^{b-{\frac {ad}{c}}}=\left(ax+{\frac {ad}{c}}\right)Q^{-\left(ax+{\frac {ad}{c}}\right)}\,}
可以用變量代換
令
t
=
a
x
+
a
d
c
{\displaystyle t=ax+{\frac {ad}{c}}}
化為
t
Q
−
t
=
a
c
Q
b
−
a
d
c
{\displaystyle tQ^{-t}={\frac {a}{c}}Q^{b-{\frac {ad}{c}}}}
即:
t
(
e
ln
Q
)
−
t
=
a
c
Q
b
−
a
d
c
{\displaystyle t\left(e^{\ln Q}\right)^{-t}={\frac {a}{c}}Q^{b-{\frac {ad}{c}}}}
同乘:
ln
Q
{\displaystyle {\ln Q}\,}
得出
t
ln
Q
⋅
e
−
t
ln
Q
=
ln
Q
⋅
a
c
Q
b
−
a
d
c
{\displaystyle t{\ln Q}\cdot e^{-t\ln Q}={\ln Q}\cdot {\frac {a}{c}}Q^{b-{\frac {ad}{c}}}}
故
t
ln
Q
=
−
W
k
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
{\displaystyle t{\ln Q}=-W_{k}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}
帶入
t
=
a
x
+
a
d
c
{\displaystyle t=ax+{\frac {ad}{c}}}
為
(
a
x
+
a
d
c
)
ln
Q
=
−
W
k
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
{\displaystyle \left(ax+{\frac {ad}{c}}\right){\ln Q}=-W_{k}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}
因此最終的解為
x
=
−
W
k
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
a
ln
Q
−
d
c
{\displaystyle x=-{\frac {W_{k}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}{a\ln Q}}-{\frac {d}{c}}}
若輔助方程:
x
e
x
=
−
a
ln
Q
c
Q
b
−
a
d
c
{\displaystyle xe^{x}=-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}}
−
a
ln
Q
c
Q
b
−
a
d
c
∈
(
−
∞
,
−
1
e
)
{\displaystyle -{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\in \left(-\infty ,-{\frac {1}{e}}\right)}
輔助方程無實數解,原方程亦無實解;
若:
−
a
ln
Q
c
Q
b
−
a
d
c
∈
{
−
1
e
}
∪
[
0
,
+
∞
)
{\displaystyle -{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\in \left\{-{\frac {1}{e}}\right\}\cup \mathbf {[} 0,+\infty )}
輔助方程有一實數解,原方程有一實解:
x
=
−
W
k
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
a
ln
Q
−
d
c
{\displaystyle x=-{\frac {W_{k}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}{a\ln Q}}-{\frac {d}{c}}}
若:
−
a
ln
Q
c
Q
b
−
a
d
c
∈
(
−
1
e
,
0
)
{\displaystyle -{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\in \left(-{\frac {1}{e}},0\right)}
輔助方程有二實解,設為
W
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
{\displaystyle W\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}
W
−
1
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
{\displaystyle {\rm {W}}_{-1}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}
為
x
1
=
−
W
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
a
ln
Q
−
d
c
{\displaystyle x_{1}=-{\frac {W\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}{a\ln Q}}-{\frac {d}{c}}}
x
2
=
−
W
−
1
(
−
a
ln
Q
c
Q
b
−
a
d
c
)
a
ln
Q
−
d
c
{\displaystyle x_{2}=-{\frac {{\rm {W}}_{-1}\left(-{\frac {a\ln Q}{c}}\,Q^{b-{\frac {ad}{c}}}\right)}{a\ln Q}}-{\frac {d}{c}}}
例子2 用類似的方法,可知以下方程的解
x
x
=
t
,
{\displaystyle x^{x}={\mathrm {t} }\,,}
為
x
=
ln
t
W
(
ln
t
)
{\displaystyle x={\frac {\ln {\rm {t}}}{W(\ln {\rm {t}})}}\,}
或
x
=
exp
(
W
k
[
ln
(
t
)
]
)
.
{\displaystyle x=\exp \left(W_{k}\left[\ln({\rm {t}})\right]\right).}
例子3 以下方程的解
x
log
b
x
=
a
{\displaystyle x\log _{b}{x}=a\,}
具有形式
x
=
a
ln
b
W
k
(
a
ln
b
)
{\displaystyle x={\frac {a{\ln b}}{W_{k}\left(a{\ln b}\right)}}}
例子4
x
a
−
b
x
=
0
{\displaystyle x^{a}-b^{x}=0\,}
a
>
0
{\displaystyle a>0\,}
b
>
0
{\displaystyle b>0\,}
x
>
0
{\displaystyle x>0\,}
取對數,
a
ln
x
=
x
ln
b
{\displaystyle a\ln x=x\ln b\,}
ln
x
x
=
ln
b
a
{\displaystyle {\frac {\ln x}{x}}={\frac {\ln b}{a}}\,}
e
ln
x
x
=
e
ln
b
a
{\displaystyle e^{\frac {\ln x}{x}}=e^{\frac {\ln b}{a}}\,}
x
1
x
=
b
1
a
{\displaystyle x^{\frac {1}{x}}=b^{\frac {1}{a}}\,}
取倒數,
(
1
x
)
1
x
=
b
−
1
a
{\displaystyle \left({\frac {1}{x}}\right)^{\frac {1}{x}}=b^{-{\frac {1}{a}}}\,}
1
x
=
−
ln
b
a
W
(
−
1
a
ln
b
)
{\displaystyle {\frac {1}{x}}=-{\frac {\ln b}{aW\left(-{\frac {1}{a}}\ln b\right)}}\,}
最終解為 :
x
=
−
a
ln
b
W
k
(
−
ln
b
a
)
{\displaystyle x=-{\frac {a}{\ln b}}W_{k}\left(-{\frac {\ln b}{a}}\right)\,}
例子5
(
a
x
+
b
)
n
=
u
c
x
+
d
{\displaystyle (ax+b)^{n}=u^{cx+d}\,}
兩邊開
n
{\displaystyle n\,}
a
{\displaystyle a\,}
x
+
b
a
=
u
c
n
x
+
d
n
a
(
cos
2
k
π
n
+
i
sin
2
k
π
n
)
{\displaystyle x+{\frac {b}{a}}={\frac {u^{{\frac {c}{n}}x+{\frac {d}{n}}}}{a}}\left(\cos {\frac {2k\pi }{n}}+{\rm {i}}\sin {\frac {2k\pi }{n}}\right)\,}
令
u
=
e
ln
u
{\displaystyle u=e^{\ln u}\,}
化為
x
+
b
a
=
e
c
ln
u
n
x
+
d
ln
u
n
a
(
cos
2
k
π
n
+
i
sin
2
k
π
n
)
{\displaystyle x+{\frac {b}{a}}={\frac {e^{{\frac {c\ln u}{n}}x+{\frac {d\ln u}{n}}}}{a}}\left(\cos {\frac {2k\pi }{n}}+{\rm {i}}\sin {\frac {2k\pi }{n}}\right)\,}
兩邊同乘
−
c
ln
u
n
u
−
c
n
x
−
c
b
n
a
{\displaystyle -{\frac {c\ln u}{n}}u^{-{\frac {c}{n}}x-{\frac {cb}{na}}}\,}
(
−
c
ln
u
n
x
−
c
b
ln
u
n
a
)
e
−
c
ln
u
n
x
−
c
b
ln
u
n
a
=
−
c
ln
u
n
a
u
d
n
−
c
b
n
a
(
cos
2
k
π
n
+
i
sin
2
k
π
n
)
{\displaystyle \left(-{\frac {c\ln u}{n}}x-{\frac {cb\ln u}{na}}\right)e^{-{\frac {c\ln u}{n}}x-{\frac {cb\ln u}{na}}}=-{\frac {c\ln u}{na}}u^{{\frac {d}{n}}-{\frac {cb}{na}}}\left(\cos {\frac {2k\pi }{n}}+{\rm {i}}\sin {\frac {2k\pi }{n}}\right)\,}
最終得
x
k
=
−
n
c
ln
u
W
k
[
−
c
ln
u
n
a
u
d
n
−
c
b
n
a
(
cos
2
k
π
n
+
i
sin
2
k
π
n
)
]
−
b
a
{\displaystyle x_{k}=-{\frac {n}{c\ln u}}W_{k}\left[-{\frac {c\ln u}{na}}u^{{\frac {d}{n}}-{\frac {cb}{na}}}\left(\cos {\frac {2k\pi }{n}}+{\rm {i}}\sin {\frac {2k\pi }{n}}\right)\right]-{\frac {b}{a}}\,}
k
∈
Z
{\displaystyle k\in {\mathbb {Z} }\,}
標準的 Lambert W 函數可用來表示以下超越代數方程式的解:
e
−
c
x
=
a
o
(
x
−
r
)
(
1
)
{\displaystyle e^{-cx}=a_{o}(x-r)~~\quad \qquad \qquad \qquad \qquad (1)}
其中 a 0 , c 與 r 為實常數。
其解為
x
=
r
+
W
(
c
e
−
c
r
a
o
)
c
{\textstyle x=r+{\tfrac {W\left({\frac {ce^{-cr}}{a_{o}}}\right)}{c}}}
Lambert W 函數之一般化[ 1] [ 2] [ 3]
一項在低維空間內廣義相對論 與量子力學 的應用(量子引力 ),實際上一種以前未知的 連結 於此二區域中,如 「Journal of Classical and Quantum Gravity」[ 4]
e
−
c
x
=
a
o
(
x
−
r
1
)
(
x
−
r
2
)
(
2
)
{\displaystyle e^{-cx}=a_{o}(x-r_{1})(x-r_{2})~~\qquad \qquad (2)}
其中 r 1 和 r 2 是不同實常數,為二維多項式的根。於此函數解有單一引數 x 但 r i 和 a o 為函數的參數。如此一來,此一般式類似於 「hypergeometric」(超幾何分布)函數與 「Meijer G「,但屬於不同類函數。當 r 1 = r 2 ,(2)的兩方可分解為 (1) 因此其解簡化為標準 W 函數。(2)式代表著 「dilaton」(軸子 )場的方程,可據此推導線性,雙體重力問題 1+1 維(一空間維與一時間維)當兩不等(靜止)質量,以及,量子力學的特徵能Delta位勢阱 給不等電位於一維空間。 量子力學的一特例特徵能的分析解三體問題,亦即(三維)氫分子離子 。[ 5] x :
e
−
c
x
=
a
o
∏
i
=
1
∞
(
x
−
r
i
)
∏
i
=
1
∞
(
x
−
s
i
)
(
3
)
{\displaystyle e^{-cx}=a_{o}{\frac {\prod _{i=1}^{\infty }(x-r_{i})}{\prod _{i=1}^{\infty }(x-s_{i})}}\qquad \qquad \qquad (3)}
其中 r i 與 s i 是相異實常數而 x 是特徵能和內核距離R之函數。式 (3) 與其特例表示於 (1) 和 (2) 是與一更大類型延遲微分方程 。由於哈代 的「虛假導數」概念,多根的特殊情況得以解決[ 6] Lambert "W" 函數於基礎物理問題之應用並未完全即使標準情況如 (1) 最近在原子,分子,與光學物理領域可見[ 7] 黎曼假設 的 Keiper-Li 準則 [ 8]
朗伯W函數在複平面上的圖像
z = Re(W0 (x + i y ))
z = Im(W0 (x + i y ))
W 函數可以用以下的遞推關係 算出:
w
j
+
1
=
w
j
−
w
j
e
w
j
−
z
e
w
j
(
w
j
+
1
)
−
(
w
j
+
2
)
(
w
j
e
w
j
−
z
)
2
w
j
+
2
{\displaystyle w_{j+1}=w_{j}-{\frac {w_{j}e^{w_{j}}-z}{e^{w_{j}}(w_{j}+1)-{\frac {(w_{j}+2)(w_{j}e^{w_{j}}-z)}{2w_{j}+2}}}}}
^ T.C. Scott and R.B. Mann, General Relativity and Quantum Mechanics: Towards a Generalization of the Lambert W Function , AAECC (Applicable Algebra in Engineering, Communication and Computing), vol. 17, no. 1, (April 2006), pp.41-47, [1] ; Arxiv [2] (頁面存檔備份 ,存於網際網路檔案館 )
^ T.C. Scott, G. Fee and J. Grotendorst, "Asymptotic series of Generalized Lambert W Function" (頁面存檔備份 ,存於網際網路檔案館 ), SIGSAM, vol. 47, no. 3, (September 2013), pp. 75-83
^ T.C. Scott, G. Fee, J. Grotendorst and W.Z. Zhang, "Numerics of the Generalized Lambert W Function" (頁面存檔備份 ,存於網際網路檔案館 ), SIGSAM, vol. 48, no. 2, (June 2014), pp. 42-56
^ P.S. Farrugia, R.B. Mann, and T.C. Scott, N-body Gravity and the Schrödinger Equation , Class. Quantum Grav. vol. 24, (2007), pp. 4647-4659, [3] ; Arxiv [4] (頁面存檔備份 ,存於網際網路檔案館 )
^ T.C. Scott, M. Aubert-Frécon and J. Grotendorst, New Approach for the Electronic Energies of the Hydrogen Molecular Ion , Chem. Phys. vol. 324, (2006), pp. 323-338, [5] (頁面存檔備份 ,存於網際網路檔案館 ); Arxiv [6] (頁面存檔備份 ,存於網際網路檔案館 )
^ Aude Maignan, T.C. Scott, "Fleshing out the Generalized Lambert W Function", SIGSAM, vol. 50, no. 2, (June 2016), pp. 45-60
^ T.C. Scott, A. Lüchow, D. Bressanini and J.D. Morgan III, The Nodal Surfaces of Helium Atom Eigenfunctions , Phys. Rev. A 75, (2007), p. 060101, [7] (頁面存檔備份 ,存於網際網路檔案館 )
^ R.C. McPhedran, T.C Scott and Aude Maignan, "The Keiper-Li Criterion for the Riemann Hypothesis and Generalized Lambert Functions", ACM Commun. Comput. Algebra, vol. 57, no. 3, (December 2023), pp. 85-110



























![{\displaystyle W(x)=\int _{-\infty }^{-{\frac {1}{e}}}{-{\frac {1}{\pi }}}\Im \left[{\frac {\rm {d}}{{\rm {d}}x}}W(x)\right]\ln \left(1-{\frac {z}{x}}\right){\rm {d}}x\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3bf1ea9825edb9dc37486b29ef3629f54f52c52c)
![{\displaystyle x\not \in \left[-{\frac {1}{e}},0\right],k\in {\mathbb {Z} }\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/53fd6c87a7b01214078d06687892fd33a9691232)


![{\displaystyle W_{k}(x)=1+\left(\ln x-1+2k\pi {\rm {i}}\right)e^{{\frac {\rm {i}}{2\pi }}\int _{0}^{\infty }\left[{\frac {1}{2}}\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}+{\rm {i}}\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\right]\cdot {\frac {{\rm {d}}t}{t+1}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/babe26f83ddb9f0591fcea00bae08e480e322f84)
![{\displaystyle {}_{W_{k}(x)=1+{\frac {\left(\ln x-1\right)\cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}-2k\pi \sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+{\rm {i}}\left[\left(\ln x-1\right)\sin {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}+2k\pi \cos {\frac {1}{4\pi }}\int _{0}^{\infty }\ln {\frac {\left(t-\ln t+\ln x\right)^{2}+\left(2k+1\right)^{2}\pi ^{2}}{\left(t-\ln t+\ln x\right)^{2}+\left(2k-1\right)^{2}\pi ^{2}}}\cdot {\frac {{\rm {d}}t}{t+1}}\right]}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }\arctan {\frac {2\pi \left(t-\ln t+\ln x\right)}{\left(t-\ln t+\ln x\right)^{2}+\left(4k^{2}-1\right)\pi ^{2}}}\cdot {\frac {\rm {{d}t}}{t+1}}}}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1bbe97f2e0426384d7058ef799b21c75d99ae587)









![{\displaystyle z\left[1+W(z)\right]{\frac {\rm {d}}{{\rm {d}}z}}W(z)=W(z)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/335f854707acfd8a0fcc7c61e0964924f072f15a)

![{\displaystyle {\frac {\rm {d}}{{\rm {d}}z}}W(z)={\frac {W(z)}{z\left[1+W(z)\right]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bd4f89b7cbdee99ee248268cf3ebda1d4171b3f5)




![{\displaystyle \int W(x){\rm {d}}x=x\left[W(x)+{\frac {1}{W(x)}}-1\right]+C}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9bc6b4621f45014de0d670d2f3c67fc3ecad49e6)












![{\displaystyle W(x)+W(y)=W\left[{\frac {xy}{W(x)}}+{\frac {xy}{W(y)}}\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3d1c5c1b56c0679636684dbde2bf7e21ed8ac788)

![{\displaystyle \Re \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\cos \left(k\arctan {\frac {x}{y}}\right)\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0d051f4afb9dbd7a1cfeca6d0db44afc98e3327a)

![{\displaystyle \Im \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\sin \left(k\arctan {\frac {x}{y}}\right)\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/80cce84264c6e3322d91899fd86de05534bfd65a)

![{\displaystyle \arg \left[W(x+y{\rm {i}})\right]=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}\arctan \left[\cot(k\arctan {\frac {x}{y}})\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fd8d40bbf60b7b82f166a0f81eb76d2b6dfbe23f)
![{\displaystyle {\overline {W(x+y{\rm {i}})}}=\sum _{k=1}^{\infty }{\frac {(-k)^{k-1}}{k!}}{\sqrt {(x^{2}+y^{2})^{k}}}\left[\cos \left(k\arctan {\frac {x}{y}}\right)-{\rm {i}}\sin \left(k\arctan {\frac {x}{y}}\right)\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2c7d6b51b9d52b3188295b5fe32a07bc8c18f0d6)














![{\displaystyle W(-{\frac {\sqrt[{7}]{8}}{7}}{\ln 2})=-{\frac {32}{7}}{\ln 2}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/88c2c31ae34096cc653a48ab1459d7e3595fda3f)


![{\displaystyle W\left(-1\right)={\frac {e^{{\frac {1}{2\pi }}\int _{0}^{\infty }{1 \over t+1}\arctan {2\pi \over t-\ln t}{\rm {d}}t}-\cos \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]+\pi \sin \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]-{\rm {i}}\left\{\pi \cos \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]+\sin \left[{\frac {1}{4\pi }}\int _{0}^{\infty }{1 \over t+1}\ln {\left(t-\ln t\right)^{2} \over 4\pi ^{2}+\left(t-\ln t\right)^{2}}{\rm {d}}t\right]\right\}}{e^{{\frac {1}{2\pi }}\int _{0}^{\infty }{1 \over t+1}\arctan {2\pi \over t-\ln t}{\rm {d}}t}}}\approx -0.31813-1.33723{\rm {i}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c240aacbf3ac467f466b19f28417ce10f6f7d6b9)

![{\displaystyle W\left[-{\frac {\ln(x+1)}{x(x+1)^{\frac {1}{x}}}}\right]=-{\frac {x+1}{x}}\ln(x+1)>,-1<x<0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbfbc0f57e483d72daadd901434f3654ec5974e4)


































![{\displaystyle x=\exp \left(W_{k}\left[\ln({\rm {t}})\right]\right).}](https://wikimedia.org/api/rest_v1/media/math/render/svg/04f2ee3ce598c11cbb8a14c20aedfbc1b2de9b99)





















![{\displaystyle x_{k}=-{\frac {n}{c\ln u}}W_{k}\left[-{\frac {c\ln u}{na}}u^{{\frac {d}{n}}-{\frac {cb}{na}}}\left(\cos {\frac {2k\pi }{n}}+{\rm {i}}\sin {\frac {2k\pi }{n}}\right)\right]-{\frac {b}{a}}\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a45d01eb537904ece465cdbec27d8b26b104ab99)