在实分析 中,由黎曼 创立的黎曼积分 (英语:Riemann integral )首次对函数 在给定区间 上的积分 给出了一个精确定义。黎曼积分在技术上的某些不足之处可由后来的黎曼-斯蒂尔杰斯积分 和勒贝格积分 得到修补。
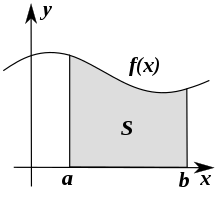
作为曲线 与坐标轴 所夹面积 的黎曼积分 让函数
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
f
(
x
)
{\displaystyle f(x)}
曲线 与
x
{\displaystyle x}
坐标轴 跟两条垂直线
x
=
a
{\displaystyle x=a}
x
=
b
{\displaystyle x=b}
面积 (既右图区域
S
{\displaystyle S}
S
{\displaystyle S}
∫
a
b
f
(
x
)
d
x
.
{\displaystyle \int _{a}^{b}f(x)\,dx.}
黎曼积分的基本概念就是对 x -轴的分割越来越细,则其所对应的矩形面积和也会越来越趋近图形
S
{\displaystyle S}
f
:
[
a
,
b
]
↦
R
<
0
{\displaystyle f:[a,b]\mapsto \mathbb {R} _{<0}}
分割越来越“细”的黎曼和。右上角的数字表示所有矩形面积(既黎曼和)。这黎曼和数列会趋于此函数的积分。 一个闭区间
[
a
,
b
]
{\displaystyle [a,b]}
分割 P
a
=
x
0
<
x
1
<
x
2
<
…
<
x
n
=
b
{\displaystyle a=x_{0}<x_{1}<x_{2}<\ldots <x_{n}=b}
[
x
i
,
x
i
+
1
]
{\displaystyle [x_{i},x_{i+1}]}
子区间 。定义
λ
{\displaystyle \lambda }
λ
=
max
(
x
i
+
1
−
x
i
)
{\displaystyle \lambda =\max(x_{i+1}-x_{i})}
0
≤
i
≤
n
−
1
{\displaystyle 0\leq i\leq n-1}
再定义取样分割 。一个闭区间
[
a
,
b
]
{\displaystyle [a,b]}
a
=
x
0
<
x
1
<
x
2
<
…
<
x
n
=
b
{\displaystyle a=x_{0}<x_{1}<x_{2}<\ldots <x_{n}=b}
[
x
i
,
x
i
+
1
]
{\displaystyle [x_{i},x_{i+1}]}
x
i
≤
t
i
≤
x
i
+
1
{\displaystyle x_{i}\leq t_{i}\leq x_{i+1}}
λ
{\displaystyle \lambda }
精细化分割 :设
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
t
0
,
…
,
t
n
−
1
{\displaystyle t_{0},\ldots ,t_{n-1}}
闭区间
[
a
,
b
]
{\displaystyle [a,b]}
y
0
,
…
,
y
m
{\displaystyle y_{0},\ldots ,y_{m}}
s
0
,
…
,
s
m
−
1
{\displaystyle s_{0},\ldots ,s_{m-1}}
0
≤
i
≤
n
{\displaystyle 0\leq i\leq n}
r
(
i
)
{\displaystyle r(i)}
x
i
=
y
r
(
i
)
{\displaystyle x_{i}=y_{r(i)}}
r
(
i
)
≤
j
<
r
(
i
+
1
)
{\displaystyle r(i)\leq j<r(i+1)}
t
i
=
s
j
{\displaystyle t_{i}=s_{j}}
y
0
,
…
,
y
m
{\displaystyle y_{0},\ldots ,y_{m}}
s
0
,
…
,
s
m
−
1
{\displaystyle s_{0},\ldots ,s_{m-1}}
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
t
0
,
…
,
t
n
−
1
{\displaystyle t_{0},\ldots ,t_{n-1}}
精细化分割 。简单来说,就是说后一个分割是在前一个分割的基础上添加一些分点和标记。
于是我们可以在此区间的所有取样分割中定义一个偏序关系 ,称作“精细”。如果一个分割是另外一个分割的精细化分割,就说前者比后者更“精细”。
对一个在闭区间
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
f
{\displaystyle f}
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
t
0
,
…
,
t
n
−
1
{\displaystyle t_{0},\ldots ,t_{n-1}}
黎曼和 (积分和 )定义为以下和式:
∑
i
=
0
n
−
1
f
(
t
i
)
(
x
i
+
1
−
x
i
)
{\displaystyle \sum _{i=0}^{n-1}f(t_{i})(x_{i+1}-x_{i})}
和式中的每一项是子区间长度
x
i
+
1
−
x
i
{\displaystyle x_{i+1}-x_{i}}
t
i
{\displaystyle t_{i}}
f
(
t
i
)
{\displaystyle f(t_{i})}
t
i
{\displaystyle t_{i}}
距离 为高,以分割的子区间为长的矩形 的面积。
不太严格地来说,黎曼积分就是当分割越来越“精细”的时候,黎曼和趋向的极限。下面的证明中,会对“越来越‘精细’”作出严格的定义。
要使得“越来越‘精细’”有效,需要把
λ
{\displaystyle \lambda }
[
x
i
,
x
i
+
1
]
{\displaystyle [x_{i},x_{i+1}]}
f
(
t
i
)
{\displaystyle f(t_{i})}
严格定义如下 :
S
{\displaystyle S}
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
ϵ
>
0
{\displaystyle \epsilon >0}
δ
>
0
{\displaystyle \delta >0}
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
t
0
,
…
,
t
n
−
1
{\displaystyle t_{0},\ldots ,t_{n-1}}
λ
≤
δ
{\displaystyle \lambda \leq \delta }
|
∑
i
=
0
n
−
1
f
(
t
i
)
(
x
i
+
1
−
x
i
)
−
S
|
<
ϵ
.
{\displaystyle \left|\sum _{i=0}^{n-1}f(t_{i})(x_{i+1}-x_{i})-S\right|<\epsilon .\,}
也就是说,对于一个函数
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
黎曼可积 的。
这个定义的缺陷是没有可操作性,因为要检验所有
λ
≤
δ
{\displaystyle \lambda \leq \delta }
另一个定义 :
S
{\displaystyle S}
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
ϵ
>
0
{\displaystyle \epsilon >0}
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
t
0
,
…
,
t
n
−
1
{\displaystyle t_{0},\ldots ,t_{n-1}}
y
0
,
…
,
y
m
{\displaystyle y_{0},\ldots ,y_{m}}
s
0
,
…
,
s
m
−
1
{\displaystyle s_{0},\ldots ,s_{m-1}}
|
∑
i
=
0
m
−
1
f
(
s
i
)
(
y
i
+
1
−
y
i
)
−
S
|
<
ϵ
.
{\displaystyle \left|\sum _{i=0}^{m-1}f(s_{i})(y_{i+1}-y_{i})-S\right|<\epsilon .\,}
这两个定义是等价的。如果有一个
S
{\displaystyle S}
S
{\displaystyle S}
λ
≤
δ
{\displaystyle \lambda \leq \delta }
δ
{\displaystyle \delta }
|
∑
i
=
0
m
−
1
f
(
s
i
)
(
y
i
+
1
−
y
i
)
−
S
|
<
ϵ
.
{\displaystyle \left|\sum _{i=0}^{m-1}f(s_{i})(y_{i+1}-y_{i})-S\right|<\epsilon .\,}
其次证明满足第二个定义的
S
{\displaystyle S}
达布积分 达布积分 。其次我们证明达布积分 的定义满足第一个定义。任选一个分割
x
0
,
…
,
x
n
{\displaystyle x_{0},\ldots ,x_{n}}
上达布和 与下达布和 都与
S
{\displaystyle S}
ϵ
2
{\displaystyle {\frac {\epsilon }{2}}}
r
{\displaystyle r}
max
0
≤
i
≤
n
−
1
(
M
i
−
m
i
)
{\displaystyle \max _{0\leq i\leq n-1}(M_{i}-m_{i})}
M
i
{\displaystyle M_{i}}
m
i
{\displaystyle m_{i}}
f
{\displaystyle f}
[
x
i
,
x
i
+
1
]
{\displaystyle [x_{i},x_{i+1}]}
上确界 和下确界 。再令
δ
{\displaystyle \delta }
ϵ
2
r
n
{\displaystyle {\frac {\epsilon }{2rn}}}
min
0
≤
i
≤
n
−
1
(
x
i
+
1
−
x
i
)
{\displaystyle \min _{0\leq i\leq n-1}(x_{i+1}-x_{i})}
δ
{\displaystyle \delta }
f
{\displaystyle f}
上达布和 或下达布和 至多相差
ϵ
2
{\displaystyle {\frac {\epsilon }{2}}}
S
{\displaystyle S}
ϵ
{\displaystyle \epsilon }
由于以上原因,黎曼积分通常被定义为达布积分(即第二个定义),因为达布积分比黎曼积分更简单、更有可操作性。
线性性:黎曼积分是线性变换 ,也就是说,如果
f
{\displaystyle f}
g
{\displaystyle g}
[
a
,
b
]
{\displaystyle [a,b]}
可积 ,
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
∫
a
b
(
α
f
+
β
g
)
d
x
=
α
∫
a
b
f
(
x
)
d
x
+
β
∫
a
b
g
(
x
)
d
x
.
{\displaystyle \int _{a}^{b}(\alpha f+\beta g)\,dx=\alpha \int _{a}^{b}f(x)\,dx+\beta \int _{a}^{b}g(x)\,dx.}
由于一个函数的黎曼积分是一个实数,因此在固定了一个区间
[
a
,
b
]
{\displaystyle [a,b]}
I
:
f
⟶
∫
a
b
f
d
x
{\displaystyle I:f\longrightarrow \int _{a}^{b}fdx}
线性泛函 。
正定性:如果函数
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
几乎处处 (勒贝格测度 意义上)大于等于0,那么它在
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
可加性:如果函数
f
{\displaystyle f}
[
a
,
c
]
{\displaystyle [a,c]}
[
c
,
b
]
{\displaystyle [c,b]}
f
{\displaystyle f}
[
a
,
b
]
{\displaystyle [a,b]}
∫
a
b
f
d
x
=
∫
a
c
f
(
x
)
d
x
+
∫
c
b
f
(
x
)
d
x
{\displaystyle \int _{a}^{b}fdx=\int _{a}^{c}f(x)\,dx+\int _{c}^{b}f(x)\,dx}
无论a 、b 、c 之间的大小关系如何,以上关系式都成立。
[
a
,
b
]
{\displaystyle [a,b]}
f
{\displaystyle f}
有界 和几乎处处 连续 的。如果
[
a
,
b
]
{\displaystyle [a,b]}
勒贝格可积 的。 如果
f
n
{\displaystyle {f_{n}}}
[
a
,
b
]
{\displaystyle [a,b]}
一致收敛 序列,其极限为
f
{\displaystyle f}
∫
a
b
f
d
x
=
∫
a
b
lim
n
→
∞
f
n
d
x
=
lim
n
→
∞
∫
a
b
f
n
d
x
.
{\displaystyle \int _{a}^{b}f\,dx=\int _{a}^{b}{\lim _{n\to \infty }{f_{n}}\,dx}=\lim _{n\to \infty }\int _{a}^{b}f_{n}\,dx.}
如果一个实函数在区间
[
a
,
b
]
,
{\displaystyle [a,b],}
单调 的,则它是黎曼可积的。 黎曼积分可推广到值属于
n
{\displaystyle n}
R
n
{\displaystyle \mathbb {R} ^{n}}
f
=
(
f
1
,
…
,
f
n
)
{\displaystyle \mathbf {f} =(f_{1},\dots ,f_{n})}
∫
f
=
(
∫
f
1
,
…
,
∫
f
n
)
{\displaystyle \int \mathbf {f} =(\int f_{1},\,\dots ,\int f_{n})}
向量空间 ,故值为复数的函数也可定义积分。
黎曼积分只定义在有界区间上,扩展到无界区间并不方便。可能最简单的扩展是通过极限来定义积分,即如同反常积分 (improper integral)一样。我们可以令
∫
−
∞
∞
f
(
t
)
d
t
=
lim
x
→
∞
∫
−
x
x
f
(
t
)
d
t
.
{\displaystyle \int _{-\infty }^{\infty }f(t)\,dt=\lim _{x\to \infty }\int _{-x}^{x}f(t)\,dt.}
不幸的是,这并不是很合适。平移不变性(如果把一个函数向左或向右平移,它的黎曼积分应该保持不变)丧失了。例如,令
f
(
x
)
=
1
{\displaystyle f(x)=1}
x
>
0
{\displaystyle x>0}
f
(
0
)
=
0
{\displaystyle f(0)=0}
f
(
x
)
=
−
1
{\displaystyle f(x)=-1}
x
<
0
{\displaystyle x<0}
x
{\displaystyle x}
∫
−
x
x
f
(
t
)
d
t
=
∫
−
x
0
f
(
t
)
d
t
+
∫
0
x
f
(
t
)
d
t
=
−
x
+
x
=
0
{\displaystyle \int _{-x}^{x}f(t)\,dt=\int _{-x}^{0}f(t)\,dt+\int _{0}^{x}f(t)\,dt=-x+x=0}
但如果我们将
f
(
x
)
{\displaystyle f(x)}
f
(
x
−
1
)
{\displaystyle f(x-1)}
x
>
1
{\displaystyle x>1}
∫
−
x
x
f
(
t
−
1
)
d
t
=
∫
−
x
1
f
(
t
−
1
)
d
t
+
∫
1
x
f
(
t
−
1
)
d
t
=
−
(
x
+
1
)
+
(
x
−
1
)
=
−
2
{\displaystyle \int _{-x}^{x}f(t-1)\,dt=\int _{-x}^{1}f(t-1)\,dt+\int _{1}^{x}f(t-1)\,dt=-(x+1)+(x-1)=-2}
由于这是不可接受的,我们可以尝试定义:
∫
−
∞
∞
f
(
t
)
d
t
=
lim
a
→
−
∞
lim
b
→
∞
∫
a
b
f
(
t
)
d
t
.
{\displaystyle \int _{-\infty }^{\infty }f(t)\,dt=\lim _{a\to -\infty }\lim _{b\to \infty }\int _{a}^{b}f(t)\,dt.}
此时,如果尝试对上面的
f
{\displaystyle f}
+
∞
{\displaystyle +\infty }
b
→
∞
{\displaystyle b\to \infty }
−
∞
{\displaystyle -\infty }
这同样也是不可接受的,我们要求积分存在且与积分顺序无关。即使这满足,依然不是我们想要的,因为黎曼积分与一致极限不再具有可交换性。例如,令
f
n
(
x
)
=
1
/
n
{\displaystyle f_{n}(x)=1/n}
[
0
,
n
]
{\displaystyle [0,n]}
n
{\displaystyle n}
∫
f
n
d
x
=
1
{\displaystyle \int f_{n}\,dx=1}
f
n
{\displaystyle f_{n}}
lim
f
n
{\displaystyle \lim f_{n}}
∫
f
d
x
≠
lim
∫
f
n
d
x
{\displaystyle \int f\,dx\not =\lim \int f_{n}\,dx}
一个更好的途径是抛弃黎曼积分而采用勒贝格积分 。虽然勒贝格积分是黎曼积分的扩展这点看上去并不是显而易见,但不难证明每个黎曼可积函数都是勒贝格可积的,并且当二者都有定义时积分值也是一致的。
事实上黎曼积分的一个直接扩展是Henstock–Kurzweil积分 。
扩展黎曼积分的另一种途径是替换黎曼累加定义中的因子
x
i
−
x
i
+
1
{\displaystyle x_{i}-x_{i+1}}
黎曼-斯蒂尔切斯积分 所采用的方法。
Shilov, G. E., and Gurevich, B. L., 1978. Integral, Measure, and Derivative: A Unified Approach , Richard A. Silverman, trans. Dover Publications. ISBN 0486635198 . 



![{\displaystyle [a,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9c4b788fc5c637e26ee98b45f89a5c08c85f7935)






![{\displaystyle f:[a,b]\mapsto \mathbb {R} _{<0}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/08f742493db4af7b3e47059a5196638caaf8ae11)

![{\displaystyle [x_{i},x_{i+1}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7138606cdb8eb7dddaba59b5aefe1ade6bc05a1b)




































![{\displaystyle [a,c]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/70b45aa26fb924cdd37786af1adcf5d58cfe15af)
![{\displaystyle [c,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/07f3f774df12e18b9f2bb94333ff320bbd2c308d)



![{\displaystyle [a,b],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2d493b840f8326ba81ff9d95b4edf1effd5f2842)



















![{\displaystyle [0,n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dcc2257be9f01b9d99b472f230da52da8aab84a8)




